NCERT/CBSE Class 6th Maths Chapter 2 Whole Numbers

NCERT/CBSE Class 6th Maths Chapter 2 Whole Numbers: NCERT Solutions for Class 6 Maths Chapter 2 Whole Numbers are comprehensive study material for students preparing for the Class 6 Mathematics exam.

NCERT/CBSE Class 6th Maths Chapter 2 Whole Numbers

• Counting numbers are called natural numbers. Natural numbers are denoted by N and given by 

N = {1, 2, 3, 4, …}

• Natural numbers included with ‘0’ are called whole numbers. Whole numbers are represented by W 

and given by W = {0, 1, 2, 3, 4, …}

• A number which comes after a given number is called its successor. 

Successor = given number + 1

• A number which comes before a given number is called its predecessor. 

Predecessor = given number – 1

• Every whole number has a successor. Every whole number except 0, has a predecessor.
• The following number line represents a whole number line on which whole numbers are represented.

The distance between two points is called unit distance.

Number operations of addition, subtraction and multiplication can easily be performed on number line.

• Closure Property for addition and multiplication: If we add or multiply two whole numbers the result is again a whole number.

Closure property does not hold good for subtraction and division of whole numbers.

• Commutativity of addition and multiplication: Whole number can be added or multiplied in any order. That is, for any two whole numbers ‘a’ and ‘b’, a + b = b + a and a × b = b × a

Commutative property does not hold good for subtraction and division of whole numbers.

• Associativity of addition and multiplication: Whole numbers can be grouped for the convenience of 

adding or multiplying. That is, for any three whole numbers ‘a’ , ‘b’ and ‘c’,a + (b + c) = (a + b) + c and a × (b × c) = (a × b) × c

Associative property does not hold good for subtraction and division of whole numbers.

• Distributivity of multiplication over addition: For any three whole numbers ‘a’ , ‘b’ and ‘c’, a × (b + c) = (a × b) + (a × c)
• Identity for addition: 0 is the identity for addition; this means when 0 is added to any whole the result is the whole number itself.
• Identity for multiplication: 1 is the identity for multiplication; this means when 1 is multiplied with any whole number the result is the whole number itself.
• Division of a whole number by zero is not defined.

Whole Numbers

The numbers 1,2, 3, are called natural numbers or counting numbers.

 Let us add one more number i.e., zero (0), to the collection of natural numbers. Now the numbers are 0,1,2, … These numbers are called whole numbers

We can say that whole nos. consist of zero and the natural numbers. Therefore, except zero all the whole nos. are natural numbers.

Facts of Whole numbers

• The smallest natural number is 1.
• The number 0 is the first and the smallest whole nos.
• There are infinitely many or uncountable number of whole-numbers.
• All natural numbers are whole-numbers.
• All whole-numbers are not natural numbers. For example, 0 is a whole-number but it is not a natural number.

The first 50 whole nos. are

0, 1, 2, 3, 4, 5, 6, 7, 8, 9, 10, 11, 12, 13,14, 15, 16, 17, 18, 19, 20, 21,

22, 23, 24, 25,26, 27, 28, 29, 30, 31, 32, 33, 34, 35, 36, 37, 38, 39, 40,

41, 42, 43, 44, 45, 46, 47, 48, 49, 50

Number line

A number line is a picture of a graduated straight and horizontal line in which numbers are written. A number written on the left-hand side of the number line is lesser and number written on the right-hand side of the number line is greater. Lets us look into some solved example problems.

In the above figure, we can see a number line where integers are placed. Here, the positive and negative integers are placed on either side of the zero. 

Find 12 × 35 using distributivity.

12 × 35 = 12 × (30 + 5)

= 12 × 30 +12 × 5

= 360 + 60 = 420.

Calculate – (2 + 3) + 4 =? = 5+ 4 = 9.

A number line is a pictorial representation of numbers on a straight line. It’s a reference for comparing and ordering numbers. It can be used to represent any real number that includes every whole number and natural number. Just to recollect, the whole number is a set of numbers that include all counting numbers (1, 2, 3,4,5,6 …….) and zero (0), whereas the natural number is the set of all counting numbers i.e. 1, 2, 3, 4, 5, 6……..

Writing numbers on a number line make it easier to compare the numbers. From the above figure, we can see that the integers on the left side are smaller than the integers on the right side. For example, 0 is less than 1, -1 is less than 0, -2 is less than -1, and so on.

Numbers on a Number Line

Arithmetic operations of numbers can be better explained on a number line. To begin with, one must know to locate numbers on a number line. Zero is the middle point of a number line. All (natural numbers) positive numbers occupy the right side of the zero whereas negative numbers occupy the left side of zero on the number line. As we move on to the left side value of a number decreases. For example, 1 is greater than -2. In a number line, integers, fractions, and decimals can also be represented easily. Check out the links given below to learn more.

Number Line 1 to 100

Here are the number lines from 1 to 100 numbers. As we already we can extend the number line indefinitely, thus students can also try to draw the line beyond 100, to practice.

Addition on Number Lines

Adding Positive Numbers

When we add two positive numbers, the result will always be a positive number. Hence, on adding positive numbers direction of movement will always be to the right side.

For example, addition of 1 and 5 (1 + 5 = 6)

Here the first number is 1 and the second number is 5; both are positive. First, locate 1 on the number line. Then move 5 places to the right will give 6.

Adding Negative Numbers

When we add two negative numbers, the result will always be a negative number. Hence, adding negative numbers direction of movement will always be to the left side.

For example, the addition of -2 and -3

Here, the first number is -2 and the second number is -3; both are negative. Locate -2 on the number line. Then move 3 places to the left will give -5.

Some other Important terms to remember

Successor	The successor of a whole number is the number obtained by adding 1 to it. Clearly, the successor of 1 is 2; successor of 2 is 3; successor of 3 is 4 and so on.
Predecessor	The predecessor of a whole number is one less than the given number. Clearly, the predecessor of 1 is 0; predecessor of 2 is 1; predecessor of 3 is 2 and so on. The whole number 0 does not have any predecessor.

Properties of Whole Numbers

Closure Property

Closure property on Addition for Whole Number

0 + 2 = 2

1 + 3 = 4

5 + 6 = 11

So Whole number are closed on Addition

Closure property on Multiplication for Whole Number

0 × 2 = 0

1 × 4 = 4

5 × 1 = 5

So Whole number are closed on Multiplication

Closure property on subtraction of Whole number

5 – 0 = 5

0 – 5 =?

1 – 3 =?

3 – 1 = 2

So Whole number are not closed on Subtraction

Closure property on Division of Whole number

21=2

12=?

02=0

20=? (Division by Zero is undefined)

So Whole Number are not closed on Division

In short

Closure Property

If a and b are any two whole numbers, then a + b, a × b are also whole numbers.

Commutative property

Commutativity property on Addition for Whole Number

So Whole number are Commutative on Addition

0 + 2 = 2 + 0 = 2

Commutativity property on Multiplication for Whole Number

0 × 2 = 0 or 2 × 0 = 0

So Whole number are Commutative on Multiplication

Commutativity property on subtraction of Whole number

5 – 0 = 5 but 0 – 5 =?

So Whole number are not Commutative on Subtraction

Commutativity property on Division of Whole number

21=2 but 12=?

So Whole Number are not Commutative on Division

In short

You can add two whole numbers in any order. You can multiply two whole numbers in

any order.

Commutative property: If a and b are any two whole numbers, then a + b = b + a and a × b = b × a

Associative property

Associativity property on Addition for Whole Number

0 + (2 + 3) = (0 + 2) + 3 = 50 + (2 + 3) = (0 + 2) + 3 = 5

1 + (2 + 3) = 6 = (1 + 2) + 31 + (2 + 3) = 6 = (1 + 2) + 3

So Whole number are Associative on Addition

Associativity property on Multiplication for Whole Number

0 × (2 × 3) = 0 or (0 × 2) × 3 = 0

So Whole number are Associative on Multiplication

Associativity property on subtraction of Whole number

10 – (2 – 1) = 9 but (10 – 2) – 1 = 7

So Whole number are not Associative on Subtraction

Associativity property on Division of Whole number

16 ÷ (4 ÷ 2) = 8 but (16 ÷ 4) ÷ 2 = 2

So Whole Number are not Associative on Division

So in Short

If a, b and c are any two whole numbers, then (a + b) + c = a + (b + c) and (a × b) × c = a × (b × c).

Distributive property

If a, b and c are any two whole numbers, then a (b + c) = a × b + a × c

Additive Identity

If a is any whole number, then a + 0 = a = 0 + a

Example

2 + 0 = 2

0 + 3 = 3

5 + 0 = 5

Multiplicative Identity

If a is any whole number, then a × 1 = a = 1 × a

Example

1 × 1 = 1

5 × 1 = 5

6 × 1 = 6

Multiplication by zero

If a is any whole number, then a × 0 = 0 = 0 × a

Example

1 × 0 = 0

5 × 0 = 0

0 × 0 = 0

Division by zero

If a is any whole number, then a ÷ 0 is not defined

Important Questions

Multiple Choice Questions:

1. What is the successor of 2001?

1. 2003
2. 2001
3. 2002
4. 2000
5. The largest 5-digit number having three different digits is:

1. 98978
2. 99897
3. 99987
4. 98799
5. The difference between 85 and the number obtained by reversing the digits is:

1. 25
2. 26
3. 27
4. 72
5. The natural numbers along with zero form the collection of:

1. Whole numbers
2. Integers
3. Rational numbers
4. Real numbers
5. Find value of 297 × 17 + 297 × 3:

1. 5940
2. 5980
3. 5942
4. 5970
5. The smallest whole number is:

1. 1
2. 0
3. not defined
4. None of these
5. Find product 12 × 35:

1. 12600
2. 34840
3. 420
4. 400
5. The difference between the smallest 3 digit number and the largest two digit number is:

1. 0
2. 1
3. 2
4. None of these
5. What is the quotient of 64 ÷ 1?

1. 1
2. 0
3. 46
4. 64
5. What are the three consecutive predecessors of 70010?

1. 70009, 70008, 7007
2. 70009, 70008, 70010
3. 70009, 70008, 70007
4. 70009
5. 3 × 10000 + 7 × 1000 + 9 × 100 + 0 × 10 + 4 is the same as:

1. 3794
2. 37940
3. 37904
4. 379409
5. Study the pattern 1 × 8 + 1 = 9

12 × 8 + 2 = 98

Next step is-

1. 123 × 8 + 3 = 987
2. 1234 × 8 + 4 = 9876
3. 120 × 8 + 3 = 963
4. 13 × 8 + 3 = 987
5. Number of whole numbers between 38 and 68 is:

1. 31
2. 30
3. 28
4. 29
5. The value of 25 × 20 × 5 is:

1. 2505
2. 2503
3. 2500
4. None of these
5. Value of 0 ÷ 5 is:

1. 5
2. 0
3. 1
4. None of these

Match The Following:

	Column I		Column II
1.	Commutative property	A.	(a × b) × c = a × (b × c)
2.	Associative Property	B.	a (b + c) = ab + ac
3.	Identity for multiplication	C.	a + b = b + a
4.	Distributive Property	D.	a × 1 = a

Fill in the blanks:

1. Division by _____ is not defined.
2. A number remains unchanged when added to ______.
3. A number remains unchanged when multiplied to ______.
4. 13 × 100 × ________ = 1300000

True/ False:

1. All natural numbers are whole numbers.
2. All whole numbers are natural numbers.
3. The predecessor of a two digit number is never a single digit number.
4. 1 is the smallest whole number.

Very Short Questions:

1. How many whole numbers are there between 32 and 53?
2. Are all whole numbers also natural numbers?
3. Complete pattern

1 1 = 1

11 11 = 121

111 111 = _______

1111 1111 = 1234321

4. Write the next three consecutive whole numbers of the following numbers:

1. 39359
2. 8632157

5. A taxi driver filled his car petrol tank with 40 litres of petrol on Monday. The next day, he filled the tank with 50 litres of petrol. If the petrol costs Rs.44 per litre, how much did he spend in all on petrol?
6. Find the product by suitable rearrangement:

1. 8 × 391 × 125
2. 2 × 1234 × 50

7. If you’re on a diet and have a breakfast consisting of 150 calories, a lunch consisting of 350 calories, and a dinner consisting of 1000 calories, then find the sum of the calories consumed that day.
8. Write the smallest whole number.
9. What is the predecessor of whole number 0?
10. Which property do the following statements hold?

(a) 6 + 4 = 4 + 6

(b) 3 + 2 = whole number

11. Add the following in three ways. Indicate the property used.

(a) 25 + 36 + 15

(b) 30 + 18 + 22

12. Using distributive property, solve the following:

(а) 360 × 102

(b) 35 × 98

13. Find the product of the greatest 3-digit number and the smallest 2-digit number.
14. Write the predecessor of the smallest 4-digit number.
15. For n = 5, verify the given statement 10 × n + 1 = n1

Short Questions:

1. Using the properties, find the values of each of the following:

(a) 736 × 102

(b) 8165 × 169 – 8165 × 69

2. Observe the following patterns and extend them by two more terms.

3. Observe the following patterns and extend them by two more terms:

4. Using the properties of whole numbers, find the value of the following in suitable way:

(a) 945 × 4 × 25

(b) 40 × 328 × 25

5. Represent the following on number line:

(a) 3 + 4

(b) 6 – 2

(c) 2 × 4

6. Give one example for each of the following properties for whole numbers.

(a) Closure property

(b) Commutative property

(c) Associative property

(d) Distributive property

7. A dealer purchased 124 LED sets. If the cost of one set is ₹38,540, determine their total cost.
8. Find the product of the greatest 3-digit number and the greatest 2-digit number.
9. Write 10 such numbers which can be shown only as line.

Long Questions:

1. 320 km distance is to be covered partially by bus and partially by train. Bus covers 180 km distance with a speed of 40 km/h and the rest of the distance is covered by the train at a speed of 70 km/h. Find the time taken by a passenger to cover the whole distance.
2. Solve the following and establish a pattern:

(a) 84 × 9

(b) 84 × 99

(c) 84 × 999

(d) 84 × 9999

3. Solve the following with suitable and short-cut method:

(a) 86 × 5

(b) 86 × 15

(c) 86 × 25

(d) 86 × 35

(e) 86 × 50

(f) 96 × 125

(g) 96 × 250

(h) 112 × 625

4. Ramesh buys 10 containers of juice from one shop and 18 containers of the same juice from another shop. If the capacity of each container is same and the cost of each of the container is ₹150, find the total money spend by Ramesh.
5. A housing complex built by DLF consists of 25 large buildings and 40 small buildings. Each large building has 15 floors with 4 apartments on each floor and each small building has 9 floors with 3 apartments on each floor. How many apartments are there in all?
6. A school principal places orders for 85 chairs and 25 tables with a dealer. Each chair cost ₹180 and each table cost ₹140. If the principal has given ₹2500 to the dealer as an advance money, then what amount to be given to the dealer now?

Assertion Reason Questions:

1. Assertion (A) – 17 is the successor of 16

Reason (R) – any natural number, you can add 1 to that number and get the next number i.e. you get its successor.

1. Both A and R are true and R is the correct explanation of A
2. Both A and R are true but R is not the correct explanation of A
3. A is true but R is false
4. A is false but R is true
5. Assertion (A) –99 is the predecessor of 100

Reason (R) – any natural number, you can substract 1 to that number and get the next number i.e. you get its predecessor.

1. Both A and R are true and R is the correct explanation of A
2. Both A and R are true but R is not the correct explanation of A
3. A is true but R is false
4. A is false but R is true

ANSWER KEY –

Multiple Choice questions:

1. C. 2002
2. C. 99987
3. C. 27
4. A. Whole numbers
5. A. 5940
6. B. 0
7. C. 420
8. B. 1
9. D. 64
10. C. 70009, 70008, 70007
11. C. 37904
12. A. 123 × 8 + 3 = 987
13. D. 29
14. C. 2500
15. B. 0

Match The Following:

	Column I		Column II
1.	Commutative property	C.	a + b = b + a
2.	Associative Property	A.	(a × b) × c = a × (b × c)
3.	Identity for multiplication	D.	a × 1 = a
4.	Distributive Property	B.	a (b + c) = ab + ac

Fill in the blanks:

1. Division by 0 is not defined.
2. A number remains unchanged when added to zero.
3. A number remains unchanged when multiplied to 1.
4. 13 × 100 × 1000 = 1300000

True /False:

1. True
2. False, every whole number except 0 is a natural number.
3. False, the predecessor of 10 is 9.
4. True

Very Short Answer:

1. There are 20 whole numbers between 32 and 53. These are 33, 34, 35, 36, 37, 38, 39, 40, 41, 42, 43, 44, 45, 46, 47, 48, 49, 50, 51 and 52.
2. No, all whole numbers are not natural numbers. (0 is a whole number but not a natural number.)
3. 12321

1. The next three consecutive whole numbers of 39359 are: 39360, 39361, 39362
2. The next three consecutive whole numbers of 8632157 are: 8632158, 8632159, 8632160.
3. Petrol filled on Monday = 40 liters.

Petrol filled the next day = 50 liters.

∴ Total petrol filled on the two days = 40 liters + 50 liters = 90 liters.

∴ Cost of petrol per liter = Rs. 44

∴ Cost of 90 liters petrol = Rs. 44 × 90 = Rs. 3960.

1. 8 × 391 × 125 = 391 × (125 × 8) = 391 × 1000 = 391,000
2. 2 × 1234 × 50 =1234 × (2 × 50) = 1234 × 100 = 123,400
3. Breakfast consisting of 150 calories.

Lunch consisting of 350 calories.

Dinner consisting of 1000 calories.

The sum of the calories consumed that day is 150 + 350 + 1000 = 1500 calories.

8. 0 is the smallest whole number.
9. Whole number 0 has no predecessor.
10. (a) 6 + 4 = 4 + 6 holds commutative property of addition.

(b) 3 + 2 = whole number holds closure property.

11. (a) 25 + 36 + 15

Way I: 25 + (36 + 15) = 25 + 51 = 76

Way II: (25 + 36) + 15 = 61 + 15 = 76

Way III: (25 + 15) + 36 = 40 + 36 = 76

Here, we have used associative property.

(b) 30 + 18 + 22

Way I: 30 + (18 + 22) = 30 + 40 = 70

Way II: (30 + 18) + 22 = 48 + 22 = 70

Way III: (30 + 22) + 18 = 52 + 18 = 70

Here, we have used associative property.

12. (a) 36 × 102 = 36 × (100 + 2) = 36 × 100 + 36 × 2 = -36000 + 72 = 36072

(b) 35 × 98 = 35 × (100 – 2) = 35 × 100 – 35 × 2 = 3500 – 70 = 3430

13. The greatest 3-digit number = 999

The smallest 2-digit number = 10

∴ Product = 999 x 10 = 9990

14. The smallest 4-digit number = 1000

∴ The predecessor of 1000 = 1000 – 1 = 999

15. Given statement is

10 x n + 1 = n1

Put n = 5, 10 x 5 + 1 = 51

⇒ 50 + 1 = 51

⇒ 51 = 51. Hence, verified.

Short Answer:

1. (a) 736 × 102 = 736 × (100 + 2)

= 736 × 100 + 736 × 2 [Using distributive property]

= 73600 + 1472 = 75072

(b) 8165 × 169 – 8165 × 69 = 8165 × (169 – 69) [Using distributive property]

= 8165 × 100 = 816500

2. Next two terms are

1010101 × 1010101= 1020304030201

101010101 × 101010101=10203040504030201

3. Next two terms are

15873 × 7 × 3 = 333333

15873 × 7 × 4 = 444444

4. (a) 945 × 4 × 25 = 945 × (4 × 25) = 945 × 100 = 94500

(b) 40 × 328 × 25 = 328 × (40 × 25) = 328 × 1000 = 328000

(a) 3 + 4

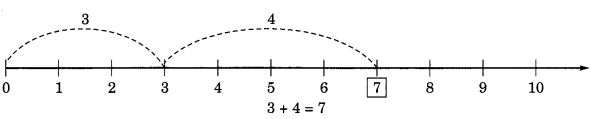
(b) 6 – 2

(c) 2 × 4

(a) 3 + 4 = 7 (whole number) closure property

(b) 4 + 5 = 5 + 4 Commutative property

(c) 3 + (5 + 7) = (3 + 5) + 7 Associative property

(d) 6 × (8 + 3) = 6 × 8 + 6 × 3 Distributive property.

7. Total cost of 124 LED sets = ₹ (38,540 × 124)

= ₹ [38,540 × (100 + 20 + 4)]

= ₹ [38,540 × 100 + 38,540 × 20 + 38,540 × 4]

= ₹ [38,54,000 + 7,70,800 + 1,54,160]

= ₹ 47,789,6

8. Greatest 3-digit number = 999

Greatest 2-digit number = 99

∴ Product = 999 × 99 = 999 × (100 – 1)

= 999 × 100 – 999 × 1

= 99900 – 999 = 98901

9. 2, 5, 7, 11, 13, 17, 19, 23, 29 and 31 are such numbers which can be shown only as line.

123 × 9 + 4 = 1111.

Long Answer:

1. Total distance = 320 km

Distance covered by the bus = 180 km

Speed of the bus = 40 km/h

∴ Time Taken by the bus =DistanceSpeed

=18040hours=92hours

Distance covered by the train = 320 – 180 = 140 km.

Speed of the train = 70 km/h

∴ Time taken by the train

=DistanceSpeed=14070 hours = 2hours

Hence, the total time taken by the passenger

= 92  hours + 2 hours

= 4 hours 30 min + 2 hours

= 6 hours 30 min

2. (a) 84 × 9 = 84 × (10 – 1) = 84 × 10 – 84 × 1 = 840 – 84 = 756

(b) 84 × 99 = 84 × (100 – 1) = 84 × 100 – 84 × 1 = 8400 – 84 = 8316

(c) 84 × 999 = 84 × (1000 – 1) = 84 × 1000 – 84 × 1 = 84000 – 84 = 83916

(d) 84 × 9999 = 84 × (10000 – 1) = 84 x 10000 – 84 × 1 = 840000 – 84 = 839916

3. (a) 86×5=86×102=43×10=430

(b) 86×15=86×302=43×30=43×10×3=430×3=1290

(c) 86×25=86×1004=43×50=43×10×5=430×5=2150

(d) 86×35=86×702=43×70=43×10×7=430×7=3010

(e) 86×50=86×1002=43×100=4300

(f) 96×125=96×10008=12×1000=12000

(g) 96×250=96×10004=24×1000=24000

(h) 112×625=112×1000016=7×10000=70000

4. Ramesh buys 10 containers from one shop Cost of 1 container = ₹ 150

He buys 18 containers of the same capacity from another shop.

Cost of 1 container = ₹150

∴ Total money spent by Ramesh

= ₹ [10 × 150 + 18 × 150]

= ₹150 × (10 + 18)

= ₹150 × 28

= ₹4200

5. Number of large buildings = 25

Number of floors = 15

Number of apartments on each floor = 4

∴ Total number of apartments in large buildings = 25 × 15 × 4

Number of small building = 40

Number of floors = 9

Number of apartments on each floor = 3

∴ Total number of apartments in small buildings = 40 × 9 × 3

Hence, the number of apartments in all = 25 × 15 × 4 + 40 × 9 × 3 = 1500 + 1080 = 2580.

6. Number of chairs = 85

Cost of one chair = ₹ 180

Cost of 85 chairs = ₹ (85 × 180)

Number of tables = 25

Cost of one table = ₹ 140

Cost of 25 tables = ₹ (25 × 140)

Total cost of all chairs and tables = ₹ (85 × 180 + 25 × 140)

= ₹ (15300 + 3500) = ₹ 18800

Money given in advance = ₹ 2500

∴ Balance money to be paid to the dealer = ₹ 18800 – ₹ 2500 = ₹ 16300

Assertion Reason Answers:

1) a) Both A and R are true and R is the correct explanation of A.2) a) Both A and R are true and R is the correct explanation of A.