NCERT Solutions For Class 6 Maths Chapter 3 Exercise 3.3

NCERT Solutions for Class 6 Maths Chapter 3: NCERT Solutions for Class 6 Maths Chapter 3 Playing With Numbers Exercise 3.3

NCERT Solutions for Class 6 Maths Chapter 3 Whole Numbers Exercise 3.3

1. Using the divisibility test, determine which of the following numbers are divisible by
2; by 3; by 4; by 5; by 6; by 8; by 9; by 10; by 11 (say, yes or no):

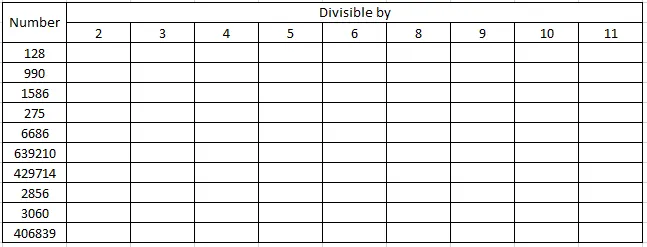
Solution:

2. Using divisibility tests, determine which of the following numbers are divisible by 4 and by 8:
(A) 572
(B) 726352
(C) 5500
(D) 6000
(E) 12159
(F) 14560
(G) 21084
(H) 31795072
(I) 1700
(J) 2150

Solution:

(A)	572	→	Divisible by 4 as its last two digits are divisible by 4.
		→	Not divisible by 8 as its last three digits are not divisible by 8.
(B)	726352	→	Divisible by 4 as its last two digits are divisible by 4.
		→	Divisible by 8 as its last three digits are divisible by 8.
(C)	5500	→	Divisible by 4 as its last two digits are divisible by 4.
		→	Not divisible by 8 as its last three digits are not divisible by 8.
(D)	6000	→	Divisible by 4 as its last two digits are 0.
		→	Divisible by 8 as its last three digits are 0.
(E)	12159	→	Not divisible by 4 and 8 as it is an odd number.
(F)	14560	→	Divisible by 4 as its last two digits are 4.
		→	Divisible by 8 as its last three digits are 8.
(G)	21084	→	Divisible by 4 as its last two digits are 4.
		→	Not divisible by 8 as its last three digits are not divisible by 8.
(H)	31795072	→	Divisible by 4 as its last two digits are divisible by 4.
		→	Divisible by 8 as its last three digits are divisible by 8.
(I)	1700	→	Divisible by 4 as its last two digits are 0.
		→	Not divisible by 8 as its last three digits are not divisible by 8.
(J)	5500	→	Not divisible by 4 as its last two digits are not divisible by 4.
		→	Not divisible by 8 as its last three digits are not divisible by 8.

3. Using divisibility tests, determine which of the following numbers are divisible by 6:
(A) 297144
(B) 1258
(C) 4335
(D) 61233
(E) 901352
(F) 438750
(G) 1790184
(H) 12583
(I) 639210
(J) 17852

Solution:

(a) 297144

Since the last digit of the number is 4. Hence, the number is divisible by 2
By adding all the digits of the number, we get 27 which is divisible by 3. Hence, the number is divisible by 3
∴ The number is divisible by both 2 and 3. Hence, the number is divisible by 6

(b) 1258

Since the last digit of the number is 8. Hence, the number is divisible by 2
By adding all the digits of the number, we get 16 which is not divisible by 3. Hence, the number is not divisible by 3
∴ The number is not divisible by both 2 and 3. Hence, the number is not divisible by 6

(c) 4335

Since the last digit of the number is 5 which is not divisible by 2. Hence, the number is not divisible by 2
By adding all the digits of the number, we get 15 which is divisible by 3. Hence, the number is divisible by 3
∴ The number is not divisible by both 2 and 3. Hence, the number is not divisible by 6

(d) 61233

Since the last digit of the number is 3 which is not divisible by 2. Hence, the number is not divisible by 2
By adding all the digits of the number, we get 15 which is divisible by 3. Hence, the number is divisible by 3
∴ The number is not divisible by both 2 and 3. Hence, the number is not divisible by 6

(e) 901352

Since the last digit of the number is 2. Hence, the number is divisible by 2
By adding all the digits of the number, we get 20 which is not divisible by 3. Hence, the number is not divisible by 3
∴ The number is not divisible by both 2 and 3. Hence, the number is not divisible by 6

(f) 438750

Since the last digit of the number is 0. Hence, the number is divisible by 2
By adding all the digits of the number, we get 27 which is divisible by 3. Hence, the number is divisible by 3
∴ The number is divisible by both 2 and 3. Hence, the number is divisible by 6

(g) 1790184

Since the last digit of the number is 4. Hence, the number is divisible by 2
By adding all the digits of the number, we get 30 which is divisible by 3. Hence, the number is divisible by 3
∴ The number is divisible by both 2 and 3. Hence, the number is divisible by 6

(h) 12583

Since the last digit of the number is 3. Hence, the number is not divisible by 2
By adding all the digits of the number, we get 19 which is not divisible by 3. Hence, the number is not divisible by 3
∴ The number is not divisible by both 2 and 3. Hence, the number is not divisible by 6

(i) 639210

Since the last digit of the number is 0. Hence, the number is divisible by 2
By adding all the digits of the number, we get 21 which is divisible by 3. Hence, the number is divisible by 3
∴ The number is divisible by both 2 and 3. Hence, the number is divisible by 6

(j) 17852

Since the last digit of the number is 2. Hence, the number is divisible by 2
By adding all the digits of the number, we get 23 which is not divisible by 3. Hence, the number is not divisible by 3
∴ The number is not divisible by both 2 and 3. Hence, the number is not divisible by 6

4. Using divisibility tests, determine which of the following numbers are divisible by 11:
(A) 5445
(B) 10824
(C) 7138965
(D) 70169308
(E) 10000001
(F) 901153

Solutions:

(a) 5445

Sum of the digits at odd places = 5 + 4 = 9
Sum of the digits at even places = 4 + 5 = 9
Difference = 9 – 9 = 0
Since the difference between the sum of digits at odd places and the sum of digits at even places is 0. Hence, 5445 is divisible by 11

(b) 10824

Sum of digits at odd places = 4 + 8 + 1 = 13
Sum of digits at even places = 2 + 0 = 2
Difference = 13 – 2 = 11
Since the difference between the sum of digits at odd places and the sum of digits at even places is 11 which is divisible by 11. Hence, 10824 is divisible by 11

(c) 7138965

Sum of digits at odd places = 5 + 9 + 3 + 7 = 24
Sum of digits at even places = 6 + 8 + 1 = 15
Difference = 24 – 15 = 9
Since the difference between the sum of digits at odd places and the sum of digits at even places is 9 which is not divisible by 11. Hence, 7138965 is not divisible by 11

(d) 70169308

Sum of digits at odd places = 8 + 3 + 6 + 0 = 17
Sum of digits at even places = 0 + 9 + 1 + 7 = 17
Difference = 17 – 17 = 0
Since the difference between the sum of digits at odd places and the sum of digits at even places is 0. Hence, 70169308 is divisible by 11

(e) 10000001

Sum of digits at odd places = 1
Sum of digits at even places = 1
Difference = 1 – 1 = 0
Since the difference between the sum of digits at odd places and the sum of digits at even places is 0. Hence, 10000001 is divisible by 11

(f) 901153

Sum of digits at odd places = 3 + 1 + 0 = 4
Sum of digits at even places = 5 + 1 + 9 = 15
Difference = 15 – 4 = 11
Since the difference between the sum of digits at odd places and the sum of digits at even places is 11 which is divisible by 11. Hence, 901153 is divisible by 11

5. Write the smallest digit and the greatest digit in the blank space of each of the following numbers so that the number formed is divisible by 3:
(A) 6724
(B) 4765__2

Solutions:

(a) __ 6724

Sum of the given digits = 19
Sum of its digit should be divisible by 3 to make the number divisible by 3
Since 21 is the smallest multiple of 3 which comes after 19
So, the smallest number = 21 – 19 = 2
Now 2 + 3 + 3 = 8
But 2 + 3 + 3 + 3 = 11
Now, if we put 8, sum of digits will be 27 which is divisible by 3
Therefore the number will be divisible by 3
Hence, the largest number is 8

(b) 4765 __ 2

Sum of the given digits = 24
Sum of its digits should be divisible by 3 to make the number divisible by 3
Since 24 is already divisible by 3. Hence, the smallest number that can be replaced is 0
Now, 0 + 3 = 3
3 + 3 = 6
3 + 3 + 3 = 9
3 + 3 + 3 + 3 = 12
If we put 9, the sum of its digits becomes 33. Since 33 is divisible by 3.
Therefore the number will be divisible by 3
Hence, the largest number is 9

6. Write the smallest digit and the largest digit in the blanks space of each of the following numbers so that the number formed is divisible by 11:
(A) 92 _ 389
(B) 8 _ 9484

(a) 92 __ 389

Let ‘a’ be placed here
Sum of its digits at odd places = 9 + 3 + 2 = 14
Sum of its digits at even places = 8 + a + 9 = 17 + a
Difference = 17 + a – 14 = 3 + a
The difference should be 0 or a multiple of 11, then the number is divisible by 11
If 3 + a = 0
a = -3
But it cannot be a negative Taking the closest multiple of 11 which is near 3
It is 11 which is near 3
Now, 3 + a = 11
a = 11 – 3
a = 8
Therefore the required digit is 8

(b) 8 __ 9484

Let ‘a’ be placed here
Sum of its digits at odd places = 4 + 4 + a = 8 + a
Sum of its digits at even places = 8 + 9 + 8 = 25
Difference = 25 – (8 + a) = 17 – a
The difference should be 0 or a multiple of 11, then the number is divisible by 11
If 17 – a = 0
a = 17 (which is not possible)
Now, take a multiple of 11.
Let’s take 11
17 – a = 11
a = 17 – 11
a = 6
Therefore, the required digit is 6